
SERVImed Industrial distribuisce a livello mondiale un portfolio di dispositivi medici oftalmici unico ed innovativo.
Mediante accordi con grandi realtà mondiali, SERVImed Industrial distribuisce a livello nazionale dispositivi medici, affiancando i produttori con le migliori strategie di vendita e marketing, contribuendo alla crescita e allo sviluppo dell’azienda e dei suoi prodotti.
SERVImed Industrial è specializzata nella realizzazione di Global Service e servizi di prevenzione, controllo e gestione delle infezioni nelle strutture sanitarie pubbliche e private.
Approfondimento sulle aziende affiliate di SERVImed Industrial SPA.
SERVImed Industrial propone un rapporto di totale partnership con Strutture ed Aziende Ospedaliere, sia in Italia che nel resto del Mondo.
SERVImed Industrial è costituita da un gruppo di professionisti, suddivisi nei seguenti dipartimenti:
Vendite | Marketing | Supporto Clinico | Customer Service | Ricerca & Sviluppo.
SERVImed Industrial nasce nel 2011 come azienda distributrice di dispositivi medici a livello nazionale, con l’obiettivo di commercializzare prodotti ad alto valore scientifico per fornire ai professionisti medici soluzioni innovative a livello pubblico e privato.
Il continuo impegno in Ricerca e Sviluppo di Servimed è testimoniato dalla continua crescita del suo portfolio prodotti e dalle nuove pubblicazioni scientifiche.
Parallelamente alla distribuzione nazionale, l’azienda lancia la linea di dispositivi medici per Oftalmologia, tramite la partnership con IROMED GROUP, azienda innovativa di ricerca, sviluppo e produzione, di cui SERVImed Industrial diventa distributore esclusivo a livello mondiale.
La mission di SERVImed Industrial è portare sul mercato formulazioni esclusive, uniche e brevettate, caratterizzate da un forte contenuto scientifico. Ciò grazie alla partnership con IROMED ed alla sua lunga esperienza in ricerca, supportata dalla produzione continua di studi clinici e pubblicazioni su riviste scientifiche internazionali ad alto impact factor.
A conferma di questo, tutti i prodotti oftalmici IROMED sono brevettati a livello internazionale e marcati CE.
Nel 2016 viene commercializzato il RIBOCROSS ® te, la prima soluzione oftalmica a base di Riboflavina e Vitamina E TPGS per cross-linking corneale.
Realizzata con formulazione brevettata ad alta penetrazione corneale, è l’unica soluzione che sfrutta le proprietà di enhancer della Vitamina E TPGS per garantire le massime prestazioni in tutti i protocolli di cross-linking corneale. RIBOCROSS ® te è l’unica soluzione utilizzabile nell’esclusivo protocollo Custom Fast CXL.

RIBOCROSS ® te, la prima soluzione oftalmica a base di Riboflavina e Vitamina E TPGS per cross-linking corneale.
SERVImed Industrial lancia CF X-LINKER ®, dispositivo UV per cross-linking corneale, che porta sul mercato il Custom Fast CXL, esclusivo protocollo customizzato in EPI-ON a basse intensità.

CF X-LINKER ®, dispositivo UV per cross-linking corneale, che porta sul mercato il Custom Fast CXL, esclusivo protocollo customizzato in EPI-ON a basse intensità.
Nel biennio successivo, SERVImed Industrial sviluppa ed espande la sua rete distributiva, stringendo accordi di partnership con realtà nazionali ed internazionali e partecipando ad eventi di livello mondiale, ritagliandosi un ruolo sempre crescente nel panorama dei dispositivi medici oftalmici, fornendo supporto clinico, tecnico e commerciale a 360°.
La linea di cross-linking viene arricchita dal RIBOFAST, soluzione con formulazione brevettata senza destrano, che allarga l’offerta commerciale di SERVImed, rendendola una tra le più versatili nel campo corneale.
Nello stesso anno nasce la linea di eye drops e viene presentato DROP defence ®, soluzione protettiva brevettata contro i raggi UV e la luce blu, prima ed unica al mondo a ricevere la certificazione come DPI.

RIBOFAST, soluzione con formulazione brevettata senza destrano, che allarga l’offerta commerciale di SERVImed, rendendola una tra le più versatili nel campo corneale.

DROP defence ®, soluzione protettiva brevettata contro i raggi UV e la luce blu, prima ed unica al mondo a ricevere la certificazione come DPI.
Il 2019 vede l’espansione del portfolio di soluzioni oftalmiche, con il lancio di DROP therapeutic ®, lacrima artificiale di alta qualità con formulazione brevettata, e DROPsept ®, unica ed innovativa soluzione con attività di protezione e riparazione.
Nello stesso anno, la partnership siglata con TECLens per l’approvazione FDA e la distribuzione di un nuovo prodotto combinato per il trattamento del cheratocono rappresenta una milestone significativa.

DROP therapeutic ®, lacrima artificiale di alta qualità con formulazione brevettata.

DROPsept ®, unica ed innovativa soluzione con attività di protezione e riparazione.
Nonostante l'incertezza causata dalla pandemia, SERVImed Industrial è riuscita a raggiungere nuovi partner e paesi nel mondo, consolidando la sua presenza globale.
Nel 2020 SERVimed Industrial lancia REmark®, innovativo colorante diagnostico a base di riboflavina, e MACUoff, il primo integratore alimentare dell'azienda.
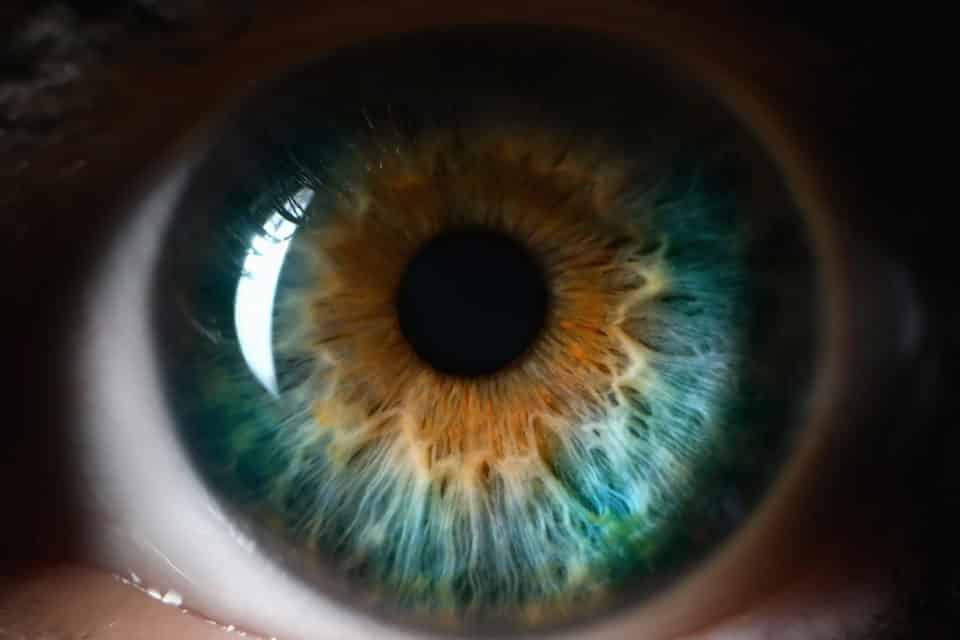
REmark ® è il primo ed unico colorante diagnostico a base di riboflavina, alternativo alla fluoresceina ed utilizzabile sia in tonometria che nella diagnosi superficiale oculare.

MACUoff ® è un integratore alimentare a base di estratti naturali e vitamine, utile per favorire e proteggere le funzionalità visive.
Tra il 2021 e il 2022 SERVImed aggiunge altri prodotti al suo portfolio oftalmico. La linea chirurgica si arricchisce con il colorante chirurgico REmark C. Le eye drops aggiungono il gel Ribohyal e la soluzione ipertonica DROPtonic 5%, mentre CLARvision si aggiunge a MACUoff per gli integratori alimentari.
Gli ultimi mesi del 2022 segnano un'importante milestone nell'espansione globale dell'azienda, con il lancio ufficiale del CF X-LINKER nel mercato Cinese.
Nel 2023 SERVImed lancia il colorante chirurgico REmark VTR-VM ed i viscoelastici Viscorib 1.0 & 1.5.

Come azienda impegnata nel settore medicale, siamo consapevoli di come un’assistenza sanitaria inadeguata comporti enormi rischi per la salute delle persone.
Ancora oggi in tantissimi paesi del “Sud del mondo” il sistema sanitario governativo non riesce a rispondere ai bisogni primari della popolazione, che si rivolgono quindi alle associazioni del terzo settore per vedere riconosciuto il loro diritto alla salute.
Abbiamo scelto di dare il nostro contributo per rafforzare la risposta di queste realtà, avvalendoci della collaborazione di Amref Health Africa, la più grande organizzazione sanitaria no profit che dal 1957 si occupa di garantire il diritto alla salute nel Continente Africano.
Una piena aderenza ai valori di Amref è stata la base di partenza di un progetto al quale siamo molto legati e che definisce in modo sostanziale il nostro contributo per restituire alla società una parte di quello che abbiamo e produciamo.
Dal 2019, infatti, contribuiremo al potenziamento e consolidamento dell’Istituto Nazionale di Formazione Sanitaria di Maridi (Maridi National Health Training Institute) in Sud Sudan, lo Stato più fragile di tutta l’Africa sub-Sahariana. Nell’ambito di questa scuola, garantiremo una borsa di studio annuale a due studenti che frequentano il corso di formazione per Operatori Sanitari di Comunità (Clinical Officer), professionisti in campo medico, capaci di svolgere circa il 75% delle attività svolte da un medico, e che costituiscono oggi l’80% della forza medica di livello intermedio di tutto il Sud Sudan.
Abbiamo scelto questo progetto perché vogliamo fornire una risposta concreta ai bisogni di questo Paese, investendo sulle sue risorse umane.
Per saperne di più, visiate il sito www.amref.it
[email protected]
Tel. +39 06 92595490
Fax +39 06 89360010
Posta certificata
[email protected]
Raffaella Broccardo | [email protected]
Marco Maria Fedeli | [email protected], [email protected]
Edoardo Menna | [email protected]
Tel. +39 337 113 84 07
Rosaria Tremamunno | [email protected]
Tel. +39 348 991 4487
Andrea Napolitano | [email protected]
Tel. +39 329 4757978
Silvia Assogna | [email protected]
Carmine Pontecorvo | [email protected]
Carmine Pontecorvo | [email protected]
Fabrizio Pieralisi | [email protected], [email protected]